I presented the Distributive Property today in my usual fashion (pun intended).
First, I tell students that they already know today’s lesson, and that they’re going to prove to me that they already know how it works.
Then I present this image (I tell them it’s from my wardrobe):
I love how this scenario never fails me – inevitably when I ask – not for the final answer, but the process and thinking that students used to find the answer, someone shares that they thought of “outfits”:
3(20 + 25)
…and someone else shares that they thought of shirts and jeans separately:
3 • 20 + 3 • 25
Lesson taught? Not quite – but it’s a great little scenario to reference as the lesson unfolds. We define the property formally and generalize using the typical a’s, b’s and c’s, and then we try an example or two. Mind you, I’m sticking with numerical expressions today – we’ll revisit this property again later once we’ve worked with like terms.
Next, I present a typical numerical example:
After we simplify the expression, I say,
“And right about now is when a student always asks me a question. Who’s got it?”
Something quite unexpected and almost magical happened next. Students had a sparkle in their eyes, almost a mischievous curiosity. This statement challenged them. And then, one by one, students started asking questions. Lots of them. It took awhile to get to the question I expected, which was, “Wait a minute – why all the arrows? Didn’t we just do “order of operations” and the whole “grouping symbols first” thing? Why this?” Instead, I was inundated with a variety of questions that told me a LOT about student thinking. I wish I would have recorded them all – I was so excited about what was happening that I can barely remember all the points they brought up. And after every question, they asked me, “Was that it? Was that THE question?” It was fun to say, “That was a GREAT question, but nope, that wasn’t it.” They ate. it. up.
When we finally got to the one question I was anticipating, students were already in a questioning frame of mind, asking,
“Wait – if we just follow “order of operations” will we still get the same answer?”
“Will we?” I put on my poker face (so hard for me!)
Everyone’s scribbling furiously, and students realize, one by one, that by Job, we DO get the same answer either way. Discussions ensued about which method students preferred, which was the perfect segue to the homework – a parallel comparison which guides students to do each problem twice – once following “order of operations” and once using the Distributive Property – then prompts students to write about which method they preferred, and why they preferred that method.
This lesson was about more than the Distributive Property…
I discovered a strategy that I’ll keep in my back pocket forever.
At any point in any lesson, couldn’t we just tell a little white lie and say, “… and right about now is when a student always asks a question… who’s got it?”
This prompt provides a safe place to question mathematics – after all, it’s about asking the question that some “other” student asked at another time in history 🙂 Somehow it made it very safe, challenging, and dare I say FUN to question mathematics today!
“Was that THE question?” 🙂


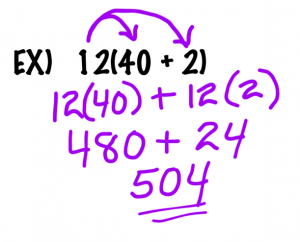















Pingback: dy/dan » Blog Archive » Great Classroom Action
I just want to say I really like this little technique and will be using it myself.
Thanks so much for your comment! I’d love to hear how it goes with students!
Pingback: Gap Filling: Multiplication | When Math Happens