Dan Meyer’s latest post got me thinking about what seems to motivate students as far as “real-world-ness” goes. What’s timely about this post is, I think some things my students did in class today go along well with reflecting upon, as Dan puts it, “theories of engagement”. How “real-world” does the math have to be to be worthwhile to kids?

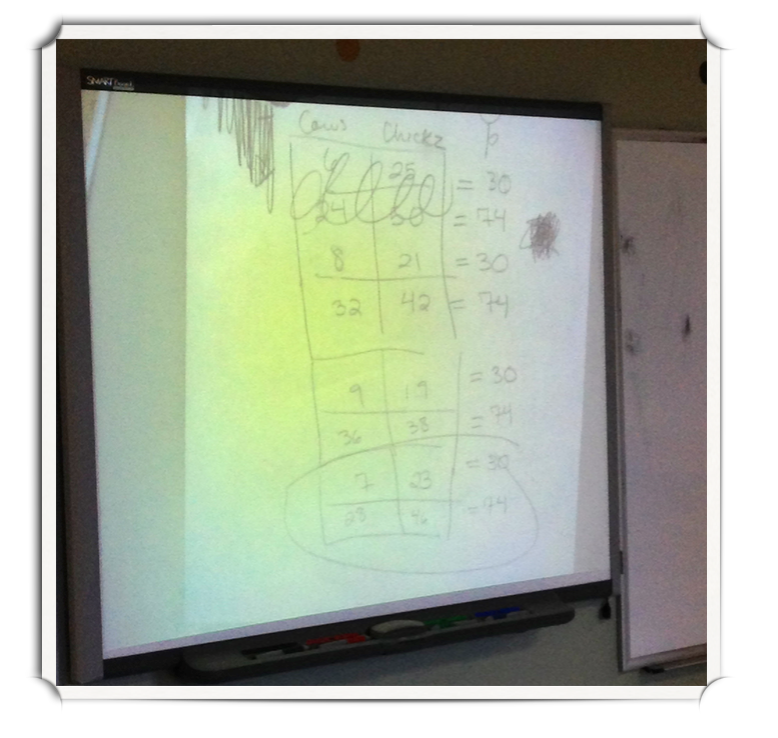
Take my algebra students, for example. Today’s objective was to “Solve Linear Systems Using Substitution.” I started class with a little novelty and my students ate it for lunch. In small groups and before any attempt at instruction on my part, students solved “The Leg Problem” by guessing-and-checking, drawing all sorts of interesting pictures, or by writing an equation that would later end up being half of our system – namely, something like 2x + 4y = 74 where x represents the number of chickens and y represents the number of cows. What’s fun for me is showing them the problem statement, which is novel but certainly not a “real-world scenario”, yet no student has EVER complained. They’re motivated enough to want to see this thing through to a solution. They rise to the challenge, start talking mathematics, and can’t wait to share and present their methods to their peers. Check out two work samples below.

My pre-algebra students started today’s lesson with a graphic I found on Facebook. It made an immediate impression. Our objective – “Finding the Percent of Change”.

Before talking about how to calculate a percent of change, I asked students to guess what they thought the percent increase was in the scenario. Their guesses are shown below.

We veered from the scenario and did some typical textbook examples… and once they got the hang of it, they were all trying to sneak back and solve the t-shirt problem. One by one, they couldn’t contain their reactions as they realized we were talking about around a 900% increase. By the time *I* was “ready to return to the t-shirt problem”, they were busting at the seams. I love acting like I didn’t know what they had been doing… I love that they couldn’t resist returning to the t-shirt problem to test their guesses. Why was it so irresistible? Maybe because it truly was “real-world” to them. Maybe it was the urge to find out just how great or how horrible their individual estimates were at the start.
All I know is, when it comes to “theories of engagement” it’s not an exact science… but when you reach that point in a lesson where the mathematics becomes irresistible, you’re in a good place. 🙂
















Pingback: In Defense of the Real World | emergent math